how to find length of a triangle given one side and angle
Eugene is a qualified control/instrumentation engineer Bsc (Eng) and has worked as a developer of electronics & software for SCADA systems.
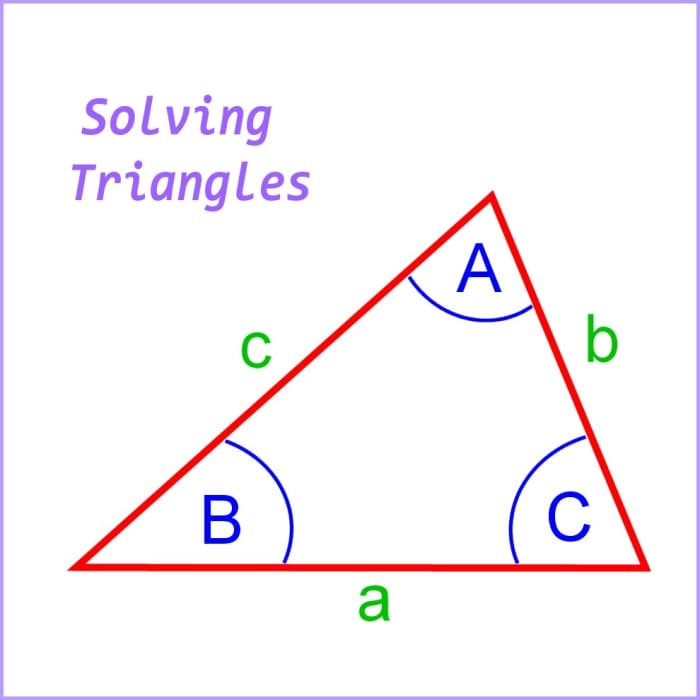
Solving triangles
© Eugene Brennan
Trigonometry and the Basics of Triangles
In this tutorial, you'll learn about trigonometry which is a branch of mathematics that covers the relationship between the sides and angles of triangles. We'll cover the basic facts about triangles first, then learn about Pythagoras's theorem, the sine rule, the cosine rule and how to use them to calculate all the angles and side lengths of triangles when you only know some of the angles or side lengths. You'll also discover different methods of working out the area of a triangle.
Please share a link to this tutorial with your friends on Pinterest, Facebook or other social media if you find it useful.
In this article, we'll discuss:
- What a Triangle Is
- Basic Facts About Triangles
- The Triangle Inequality Theorem
- Different Types of Triangles
- Using the Greek Alphabet for Equations
- Finding the Sides and Angles of a Triangle
- The Pythagorean Theorem
- Measuring Angles
- Sine, Cosine and Tangent
- Getting the Area of a Triangle
- FAQs
What Is a Triangle?
By definition, a triangle is a polygon with three sides.
Polygons are plane shapes with several straight sides. "Plane" just means they're flat and two-dimensional. Other examples of polygons include squares, pentagons, hexagons and octagons. The word plane originates from the Greek polús meaning "many" and gōnía meaning "corner" or "angle." So polygon means "many corners." A triangle is the simplest possible polygon, having only three sides.

Polygons with different numbers of sides. Regular polgons have sides the same length.
© Eugene Brennan
Basic Facts About Triangles
The most basic fact about triangles is that all the angles add up to a total of 180 degrees. The angle between the sides can be anything from greater than 0 to less than 180 degrees. The angles can't be 0 or 180 degrees, because the triangles would become straight lines. (These are called degenerate triangles).
Degrees can be written using the symbol º. So, 45º means 45 degrees.
Triangles come in many shapes and sizes according to the angles of their corners. Some triangles, called similar triangles, have the same angles but different side lengths. This changes the ratio of the triangle, making it bigger or smaller, without changing the degree of its three angles.
Below, we will examine the many ways to discover the side lengths and angles of a triangle.
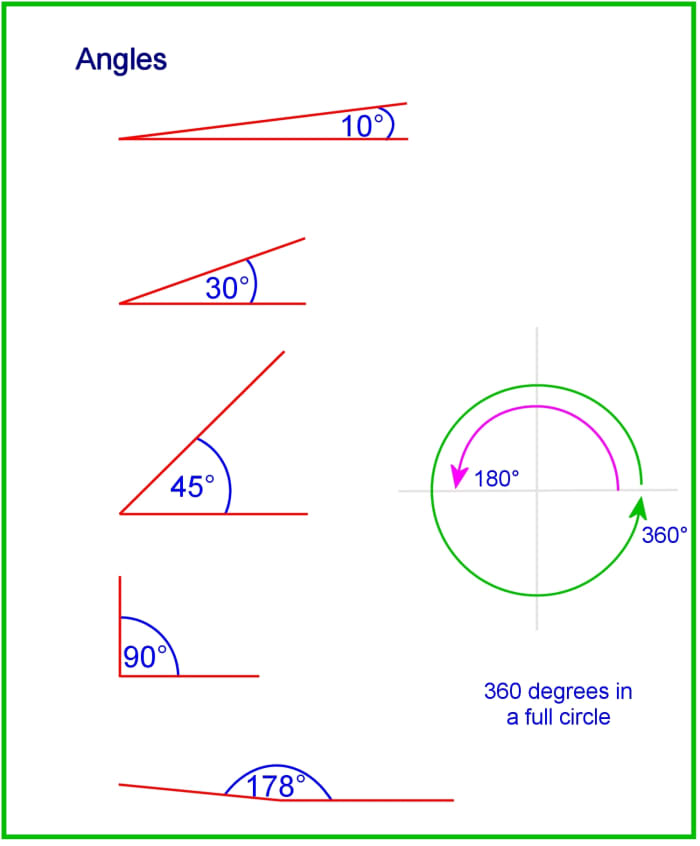
Angles of a triangle range from 0 to less than 180 degrees.
© Eugene Brennan
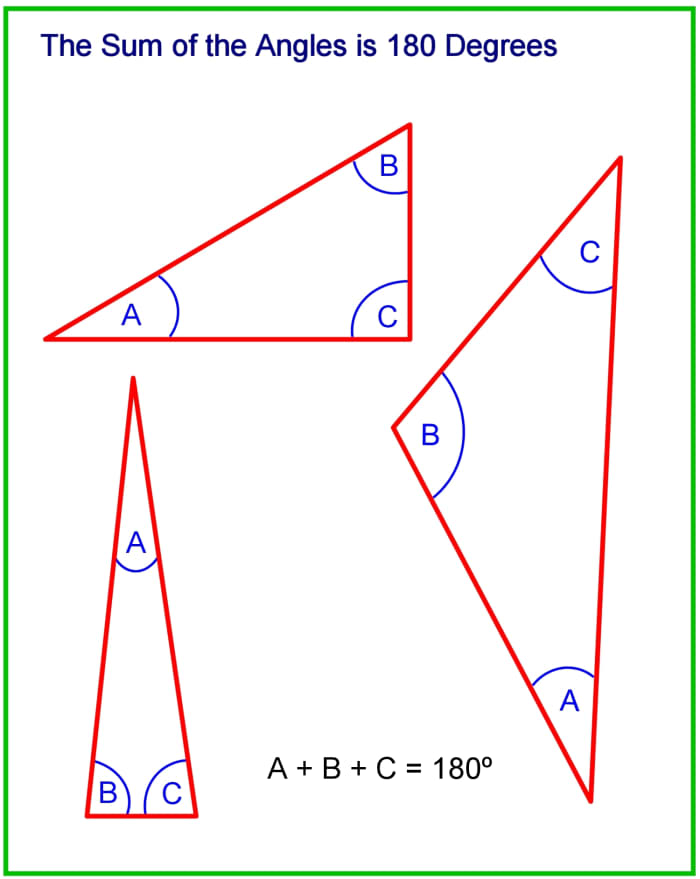
No matter what the shape or size of a triangle, the sum of the 3 angles is 180
© Eugene Brennan

Similar triangles.
© Eugene Brennan
What Is the Triangle Inequality Theorem?
This states that the sum of any two sides of a triangle must be greater than or equal to the remaining side.
What Are the Different Types of Triangles?
Before we learn how to work out the sides and angles of a triangle, it's important to know the names of the different types of triangles. The classification of a triangle depends on two factors:
- The length of a triangle's sides
- The angles of a triangle's corners
Below is a graphic and table listing the different types of triangles along with a description of what makes them unique.
Types of Triangles By Sides and Angles
You can classify a triangle either by side length or internal angle.
| Type of Triangle by Lengths of Sides | Description |
|---|---|
| Isosceles | An isosceles triangle has two sides of equal length, and one side that is either longer or shorter than the equal sides. Angle has no bearing on this triangle type. |
| Equilateral | All sides and angles are equal in length and degree. |
| Scalene | All sides and angles are of different lengths and degrees. |
| Type of Triangle by Internal Angle | Description |
|---|---|
| Right (right angled) | One angle is 90 degrees. |
| Acute | Each of the three angles measure less than 90 degrees. |
| Obtuse | One angle is greater than 90 degrees. |
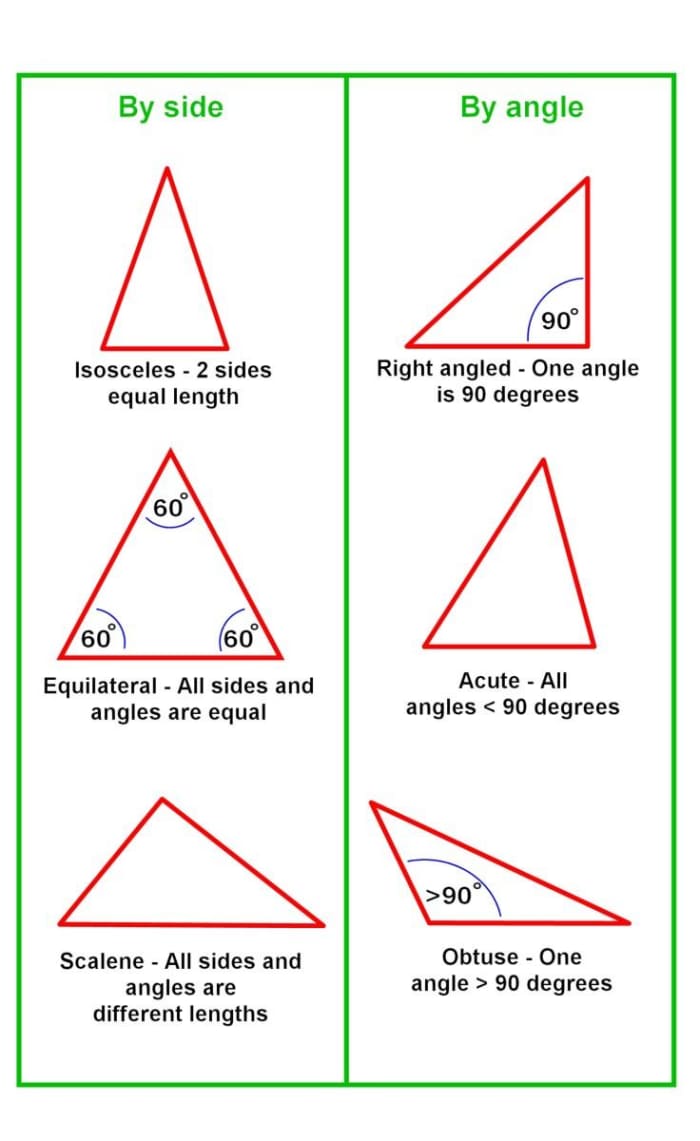
Triangles classified by side and angles.
© Eugene Brennan
Using the Greek Alphabet for Equations
Another topic that we'll briefly cover before we delve into the mathematics of solving triangles is the Greek alphabet.
In science, mathematics, and engineering many of the 24 characters of the Greek alphabet are borrowed for use in diagrams and for describing certain quantities.
You may have seen the character μ (mu) represent micro as in micrograms μg or micrometers μm. The capital letter Ω (omega) is the symbol for ohms in electrical engineering. And, of course, π (pi) is the ratio of the circumference to the diameter of a circle.
In trigonometry, the characters θ (theta) and φ (phi) are often used for representing angles.
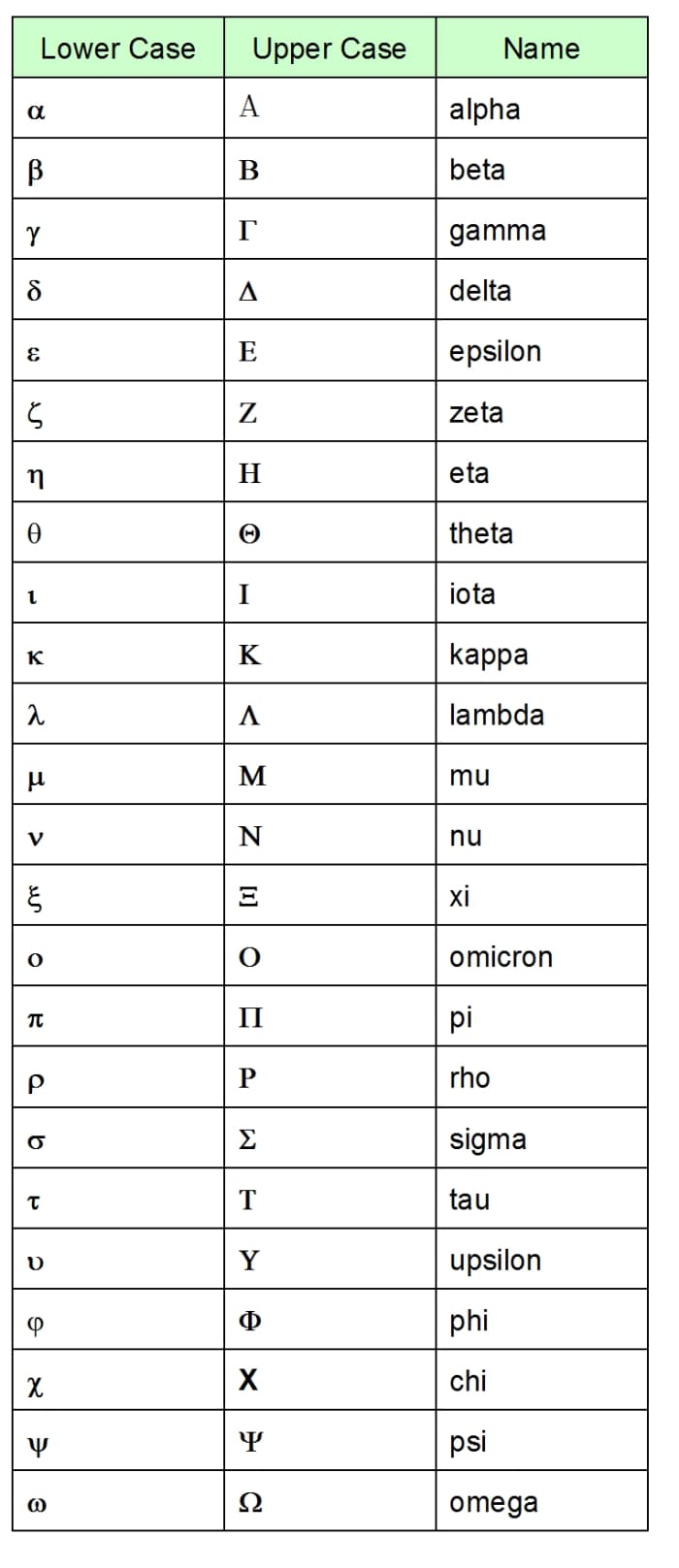
Letters of the Greek alphabet.
© Eugene Brennan
How Do You Find the Sides and Angles of a Triangle?
There are many methods available when it comes to discovering the sides and angles of a triangle. To find the length or angle of a triangle, one can use formulas, mathematical rules, or the knowledge that the angles of all triangles add up to 180 degrees.
Tools to Discover the Sides and Angles of a Triangle
- Pythagoras's theorem
- Sine rule
- Cosine rule
- The fact that all angles add up to 180 degrees
Pythagoras's Theorem (The Pythagorean Theorem)
Pythagoras's theorem uses trigonometry to discover the longest side (hypotenuse) of a right triangle (right angled triangle in British English). It states that for a right triangle:
The square on the hypotenuse equals the sum of the squares on the other two sides.
If the sides of a triangle are a, b and c and c is the hypotenuse, Pythagoras's Theorem states that:
c 2 = a 2 + b 2
c = √(a2 + b 2)
The hypotenuse is the longest side of a right triangle, and is located opposite the right angle.
So, if you know the lengths of two sides, all you have to do is square the two lengths, add the result, then take the square root of the sum to get the length of the hypotenuse.

Pythagoras's Theorem
© Eugene Brennan
Example Problem Using the Pythagorean Theorem
The sides of a triangle are 3 and 4 units long. What is the length of the hypotenuse?
Call the sides a, b, and c. Side c is the hypotenuse.
a = 3
b = 4c = Unknown
So, according to the Pythagorean theorem:
c2 = a2 + b 2
So, c2 = 32 + 42 = 9 + 16 = 25
c = √25
c = 5
How Do You Measure Angles?
You can use a protractor or a digital angle finder like this one from Amazon. These are useful for DIY and construction if you need to measure an angle between two sides, or transfer the angle to another object. You can use this as a replacement for a bevel gauge for transferring angles e.g. when marking the ends of rafters before cutting. The rules are graduated in inches and centimetres and angles can be measured to 0.1 degrees.
Note that this isn't suitable as a technical drawing instrument because the hub won't sit flat on paper unlike a protractor. Also since it's made of stainless steel, it has pointed corners which may be sharp and therefore isn't suitable for young children.

You can draw and measure angles with a protractor.
© Eugene Brennan
Sine, Cosine and Tangent of an Angle
A right triangle has one angle measuring 90 degrees. The side opposite this angle is known as the hypotenuse (another name for the longest side). The length of the hypotenuse can be discovered using Pythagoras's theorem, but to discover the other two sides, sine and cosine must be used. These are trigonometric functions of an angle.
In the diagram below, one of the angles is represented by the Greek letter θ. (pronounced "the - ta"). Side a is known as the "opposite" side and side b is called the "adjacent" side because of their positions relative to the angle θ.
The vertical lines "||" around the words below mean "length of."
So sine, cosine and tangent are defined as follows:
sine θ = |opposite side| / |hypotenuse|
cosine θ = |adjacent side| / |hypotenuse|
tan θ = |opposite side| / |adjacent side|
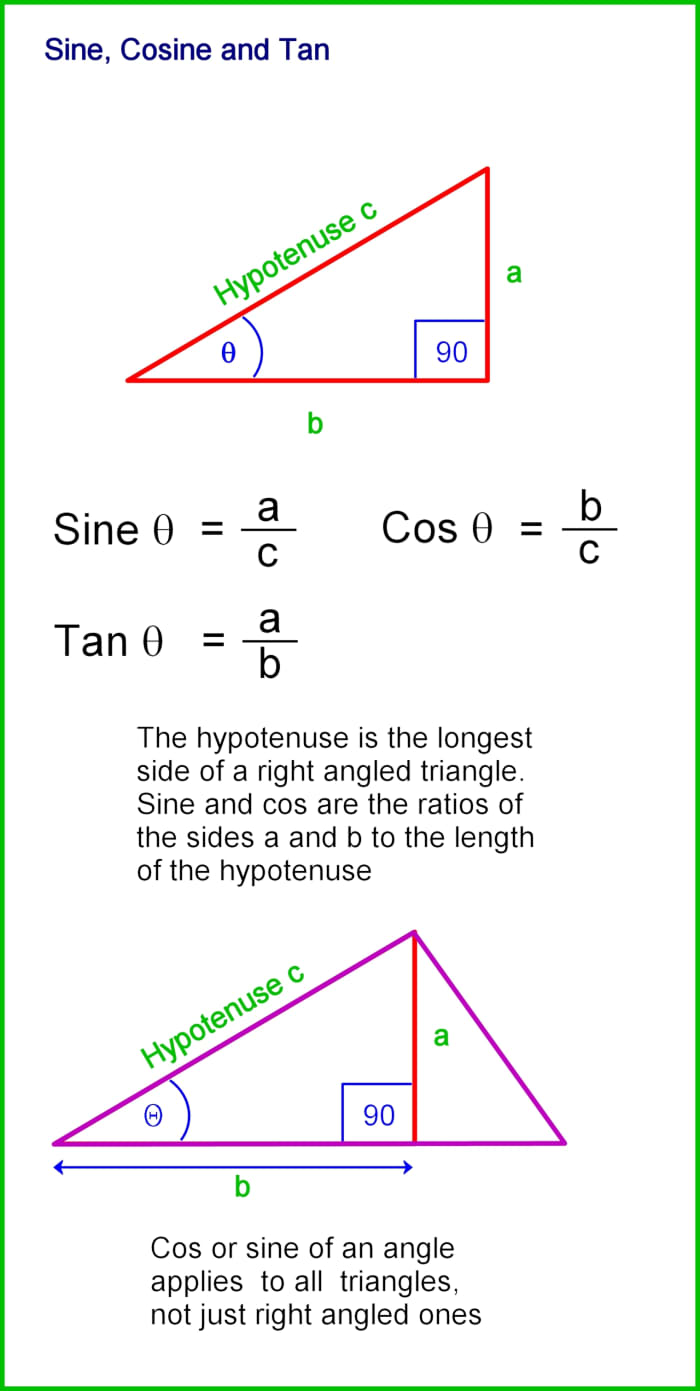
Sine, cosine and tan.
© Eugene Brennan
Sine and cosine apply to an angle, any angle, so it's possible to have two lines meeting at a point and to evaluate sine or cosine for that angle even though there's no triangle as such. However, sine and cosine are derived from the sides of an imaginary right triangle superimposed on the lines.
For instance, in the second diagram above, the purple triangle is scalene not right angled. However, you can imagine a right-angled triangle superimposed on the purple triangle, from which the opposite, adjacent and hypotenuse sides can be determined.
Over a range 0 to 90 degrees, sine ranges from 0 to 1, and cosine ranges from 1 to 0.
Remember, sine and cosine only depend on the angle, not the size of the triangle. So if the length a changes in the diagram above when the triangle changes in size, the hypotenuse c also changes in size, but the ratio of a to c remains constant. They are similar triangles.
Sine, cosine and tangent are often abbreviated to sin, cos and tan respectively.
The Sine Rule
The ratio of the length of a side of a triangle to the sine of the angle opposite is constant for all three sides and angles.
So, in the diagram below:
a / sine A = b / sine B = c / sine C
Now, you can check the sine of an angle using a scientific calculator or look it up online. In the old days before scientific calculators, we had to look up the value of the sine or cos of an angle in a book of tables.
The opposite or reverse function of sine is arcsine or "inverse sine", sometimes written as sin-1 . When you check the arcsine of a value, you're working out the angle which produced that value when the sine function was operated on it. So:
sin (30º) = 0.5 and sin -1 (0.5) = 30º
When Should the Sine Rule Be Used?
The length of one side and the magnitude of the angle opposite is known. Then, if any of the other remaining angles or sides are known, all the angles and sides can be worked out.
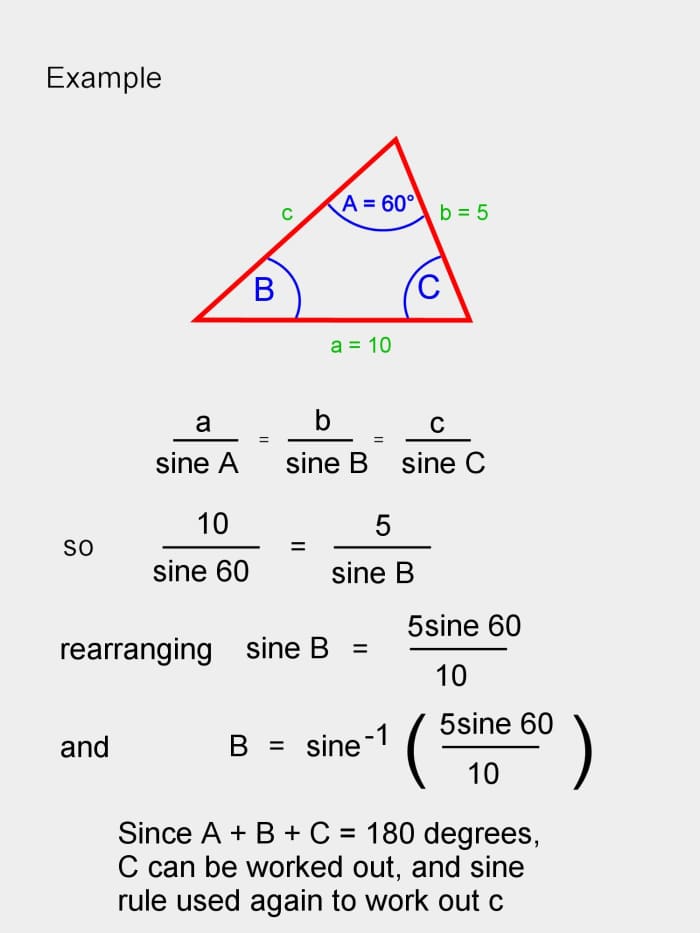
Example showing how to use the sine rule to calculate the unknown side c.
© Eugene Brennan
The Cosine Rule
For a triangle with sides a, b, and c, if a and b are known and C is the included angle (the angle between the sides), C can be worked out with the cosine rule. The formula is as follows:
c = a 2 + b 2 - 2ab cos C
When Should the Cosine Rule Be Used?
- You know the lengths of the two sides of a triangle and the included angle. You can then work out the length of the remaining side using the cosine rule.
- You know all the lengths of the sides but none of the angles.
Then, by rearranging the cosine rule equation:
C = arccos ((a 2 + b 2 - c 2) / 2ab)
The other angles can be worked out similarly.
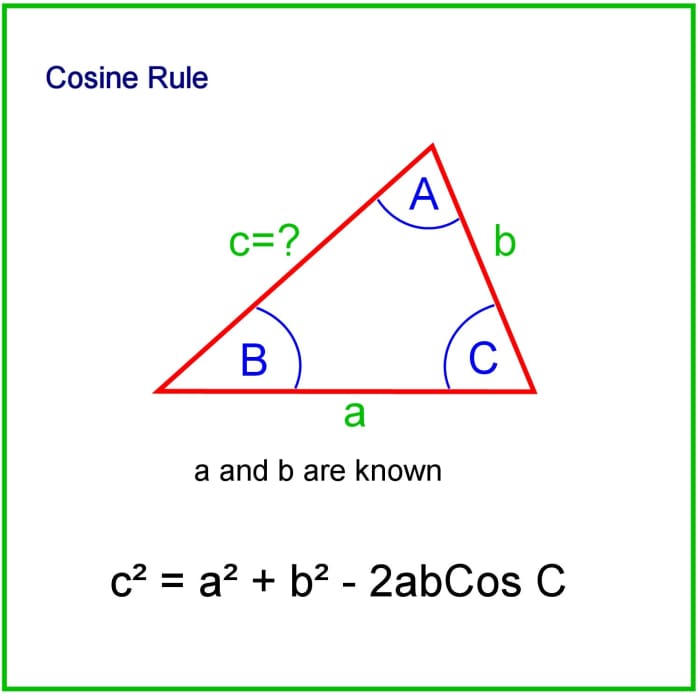
The cosine rule.
© Eugene Brennan
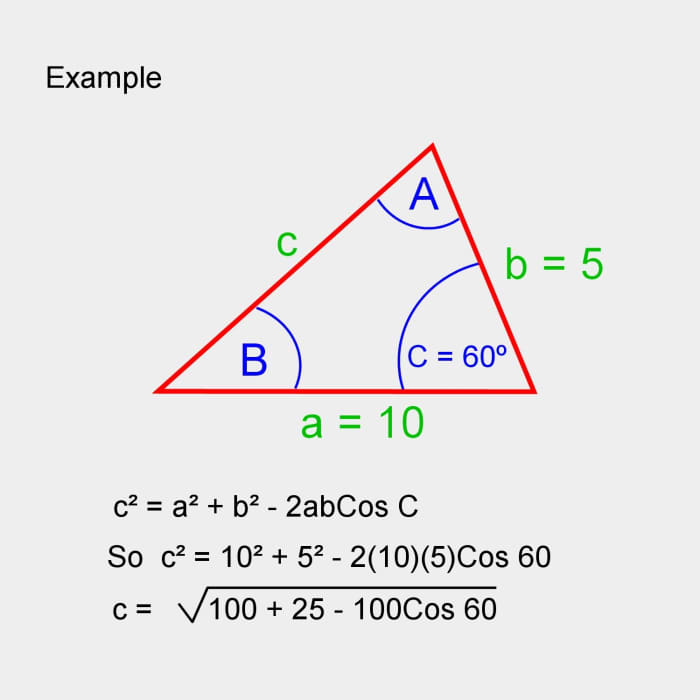
Example using the cosine rule.
© Eugene Brennan
How to Find the Angles of a Triangle Knowing the Ratio of the Side Lengths
If you know the ratio of the side lengths, you can use the cosine rule to work out two angles then the remaining angle can be found knowing all angles add to 180 degrees.
Example:
A triangle has sides in the ratio 5:7:8. Find the angles.
Answer:
So call the sides a, b and c and the angles A, B and C and assume the sides are a = 5 units, b = 7 units and c = 8 units. It doesn't matter what the actual lengths of the sides are because all similar triangles have the same angles. So if we work out the values of the angles for a triangle which has a side a = 5 units, it gives us the result for all these similar triangles.
Use the cosine rule. So c 2 = a 2 + b 2 - 2ab cos C
Substitute for a,b and c giving:
8² = 5² + 7² - 2(5)(7) cos C
Working this out gives:
64 = 25 + 49 - 70 cos C
Simplifying and rearranging:
cos C = 1/7 and C = arccos(1/7).
You can use the cosine rule again or sine rule to find a second angle and the third angle can be found knowing all the angles add to 180 degrees.
How to Get the Area of a Triangle
There are three methods that can be used to discover the area of a triangle.
Method 1. Using the Perpendicular Height
The area of a triangle can be determined by multiplying half the length of its base by the perpendicular height. Perpendicular means at right angles. But which side is the base? Well, you can use any of the three sides. Using a pencil, you can work out the area by drawing a perpendicular line from one side to the opposite corner using a set square, T-square, or protractor (or a carpenter's square if you're constructing something). Then, measure the length of the line and use the following formula to get the area:
Area = 1/2ah
"a" represents the length of the base of the triangle and "h" represents the height of the perpendicular line.
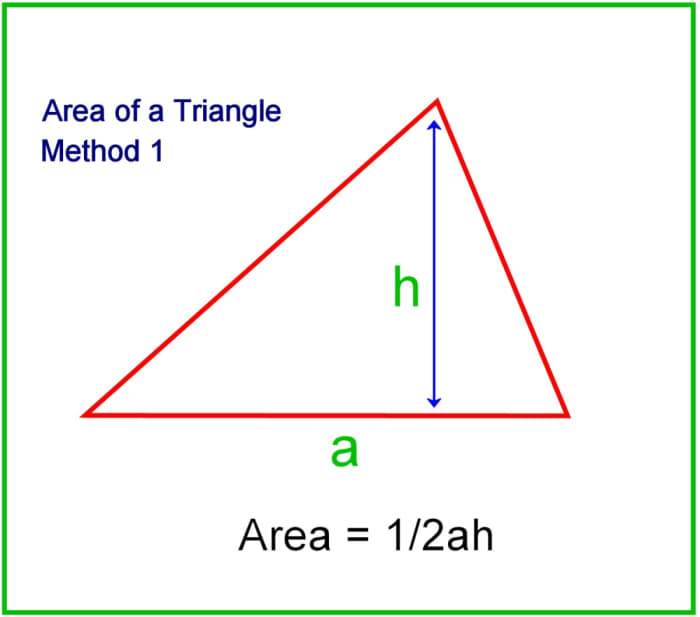
Working out the area of a triangle from the base lengtth and perpendicular height.
© Eugene Brennan
Method 2. Using Side Lengths and Angles
The simple method above requires you to actually measure the height of a triangle. If you know the length of two of the sides and the included angle, you can work out the area analytically using sine and cosine (see diagram below).
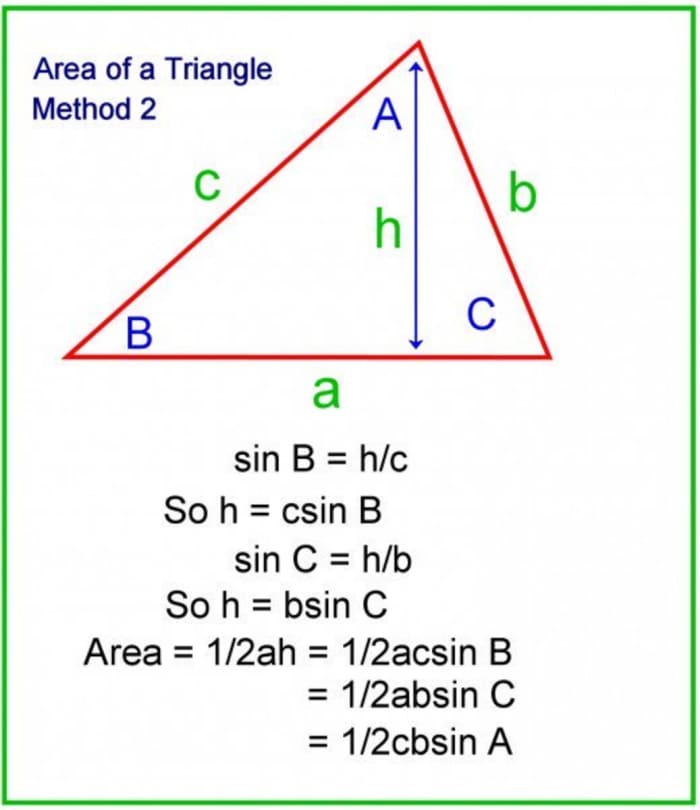
Working out the area of a triangle from the lengths of two sides and the sine of the included angle.
© Eugene Brennan
Method 3. Use Heron's Formula
All you need to know are the lengths of the three sides.
Area = √(s(s - a)(s - b)(s - c))
Where s is the semiperimeter of the triangle
s = (a + b + c)/2

Using Heron's formua to work out the area of a triangle.
© Eugene Brennan
Summary
If you've made it this far, you've learned numerous helpful methods to discover different aspects of a triangle. With all this information, you may be confused as to when you should use which method. The table below should help you identify which rule to use depending on the parameters you have been given.
Find the Angles and Sides of a Triangle: Which Rule Do I Use?
| Known Parameters | Triangle Type | Rule to Use |
|---|---|---|
| Triangle is right and I know length of two sides. | SSS after Pythagoras's Theorem used | Use Pythagoras's Theorem to work out remaining side and sine rule to work out angles. |
| Triangle is right and I know the length of one side and one angle | AAS after third angle worked out | Use the trigonometric identities sine and cosine to work out the other sides and sum of angles (180 degrees) to work out remaining angle. |
| I know the length of two sides and the angle between them. | SAS | Use the cosine rule to work out remaining side and sine rule to work out remaining angles. |
| I know the length of two sides and the angle opposite one of them. | SSA | Use the sine rule to work out remaining angles and side. |
| I know the length of one side and all three angles. | AAS | Use the sine rule to work out the remaining sides. |
| I know the lengths of all three sides | SSS | Use the cosine rule in reverse to work out each angle. C = Arccos ((a² + b² - c²) / 2ab) |
| I know the length of a side and the angle at each end | AAS | Sum of three angles is 180 degrees so remainging angle can be calculated. Use the sine rule to work out the two unknown sides |
| I know the length of a side and one angle | You need to know more information, either one other side or one other angle. Thes exception is if the known angle is in a rightangled triangle and not the right angle. |
FAQs About Triangles
Below are some frequently asked questions about triangles.
What Do the Angles of a Triangle Add Up to?
The interior angles of all triangles add up to 180 degrees.
What Is the Hypotenuse of a Triangle?
The hypotenuse of a triangle is its longest side.
What Do the Sides of a Triangle Add up to?
The sum of the sides of a triangle depends on the individual lengths of each side. Unlike the interior angles of a triangle, which always add up to 180 degrees
How Do You Calculate the Area of a Triangle?
To calculate the area of a triangle, simply use the formula:
Area = 1/2ah
"a" represents the length of the base of the triangle. "h" represents its height, which is discovered by drawing a perpendicular line from the base to the peak of the triangle.
How Do You Find the Third Side of a Triangle That Is Not Right?
If you know two sides and the angle between them, use the cosine rule and plug in the values for the sides b, c, and the angle A.
Next, solve for side a.
Then use the angle value and the sine rule to solve for angle B.
Finally, use your knowledge that the angles of all triangles add up to 180 degrees to find angle C.
How Do You Find the Missing Side of a Right Angled Triangle?
Use the Pythagorean theorem to find the missing side of a triangle. The formula is as follows:
c2 = a2 + b 2
c = √(a 2 + b 2)
What Is the Name of a Triangle With Two Equal Sides?
A triangle with two equal sides and one side that is longer or shorter than the others is called an isosceles triangle.
What Is the Cosine Formula?
This formula gives the square on a side opposite an angle, knowing the angle between the other two known sides. For a triangle, with sides a, b and c and angles A, B and C the three formulas are:
a 2 = b 2 + c 2 - 2bc cos A
or
b 2 = a 2 + c 2 - 2ac cos B
or
c 2 = a 2 + b 2 - 2ab cos C
How to Figure Out the Sides of a Triangle if I Know All the Angles?
You need to know at least one side, otherwise, you can't work out the lengths of the triangle. There's no unique triangle that has all angles the same. Triangles with the same angles are similar but the ratio of sides for any two triangles is the same.
How to Work Out the Sides of a Triangle if I know All the Sides?
Use the cosine rule in reverse.
The cosine rule states:
c 2 = a 2 + b 2 - 2ab cos C
Then, by rearranging the cosine rule equation, you can work out the angle
C = arccos ((a 2 + b 2 - c 2) / 2ab)
andB
= arccos ((a 2+ c 2 - b 2) / 2ac)
The third angle A is (180 - C - B)
How to Find the Perimeter of a Triangle
Finding the perimeter of a triangle is a straightforward operation. The perimeter is equivalent to the added lengths of all three sides.
perimeter = a + b + c
How to Find the Height of a Triangle
Finding the height of a triangle is easy if you have the triangle's area. If you're given the area of the triangle:
height = 2 x area / base
If you don't have the area, but only have the side lengths of the triangle, use the following:
height = 0.5 x √ ((a + b + c)(-a + b + c)(a - b + c)(a + b - c)) / b
If you only have two sides and the angle between them, try this formula:
area = 0.5 (a)(b)(sin(γ)), then
height = area(sin(γ))
Triangles in the Real World
A triangle is the most basic polygon and can't be pushed out of shape easily, unlike a square. If you look closely, triangles are used in the designs of many machines and structures because the shape is so strong.
The strength of the triangle lies in the fact that when any of the corners are carrying weight, the side opposite acts as a tie, undergoing tension and preventing the framework from deforming. For example, on a roof truss, the horizontal ties provide strength and prevent the roof from spreading out at the eaves.
The sides of a triangle can also act as struts, but in this case, they undergo compression. An example is a shelf bracket or the struts on the underside of an airplane wing or the tail wing itself.

How to Implement the Cosine Rule in Excel
You can implement the cosine rule in Excel using the ACOS Excel function to evaluate arccos. This allows the included angle to be worked out, knowing all three sides of a triangle.
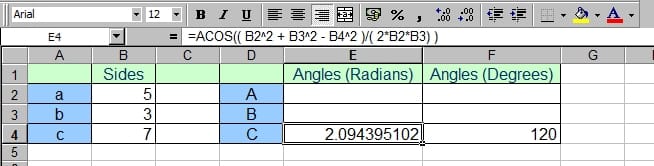
Using the Excel ACOS function to work out an angle, knowing three sides of a triangle. ACOS returns a value in radians.
© Eugene Brennan
How to Calculate Arc Length of a Circle, Segment and Sector Area
This content is accurate and true to the best of the author's knowledge and is not meant to substitute for formal and individualized advice from a qualified professional.
Questions & Answers
Question: How do you find the remaining sides of a triangle if you have only one angle and one side given?
Answer: You need to have more information. So either one side and the two angles at each end or two sides and the angle between them.
You can prove this to yourself by drawing out the single side and angle and seeing how you can draw as many different shaped triangles as you want.
Question: How do I find the value if all three sides of a scalene triangle are unknown?
Answer: If all the sides are unknown, you can't solve the triangle. You need to know at least two angles and one side, or two sides and one angle, or one side and one angle if the triangle is a right-angled triangle.
Question: What is the formula for finding what an equilateral triangle of side a, b and c is?
Answer: Since the triangle is equilateral, all the angles are 60 degrees. However, the length of at least one side must be known. Once you know that length, since the triangle is equilateral, you know the length of the other sides because all sides are of equal length.
Question: How would you solve this problem: The angle of elevation of the top of a tree from point P due west of the tree is 40 degrees. From a second point Q due east of the tree, the angle of elevation is 32 degrees. If the distance between P and Q is 200m, find the height of the tree, correct to four significant figures?
Answer: One angle is 40 degrees, the other angle is 32 degrees, therefore the third angle opposite the base PQ is 180 - (32 + 40) = 108 degrees.
You know one side of the triangle has length PQ = 200 m
A right angled triangle is formed between point P, the top of the tree and its base and also point Q, the top of the tree and its base.
The best way to solve is to find the hypotenuse of one of the triangles.
So use the triangle with vertex P.
Call the point at the top of the tree T
Call the height of the tree H
The angle formed between sides PT and QT was worked out as 108 degrees.
Using the Sine Rule, PQ / Sin(108) = PT/ Sin(32)
So for the right angled triangle we chose, PT is the hypotenuse.
Rearranging the equation above
PT = PQSin(32) / Sin(108)
Sin(40) = H / PT
So H = PTSin(40)
Substituting the value for the hypotenuse PT we calculated above gives
H = (PQSin(32) / Sin(108)) x Sin(40)
= PQSin(32)Sin(40)/Sin(108)
= 71.63 m
Question: How do I find the missing side of a triangle when only its height is known?
Answer: Use Pythagoras's Theorem. Add the sine, cosine and tan relationships between angles and the hypotenuse of the triangle to work out the remaining side.
Question: How do you find the length of all the sides of a right triangle if all you know is Cos B is 0.75?
Answer: You can find the angle B from the arccos of 0.75 and then use the fact that the three angles add up to 180 to find the remaining angle. However there is an infinite number of similar right triangles that have all three angles the same, so you need to know at least the length of one side.
Question: Which formula is used when given 90-degree triangle, opposite angle is 26 degrees and one leg is know?
Answer: Use the fact that the cos of an angle is the length of the adjacent side divided by the hypotenuse, or the sine of an angle is the opposite side divided by the hypotenuse. In your case, you know the side opposite the angle.
So sine (26 degrees) = length opposite side / length hypotenuse
Therefore
Length hypotenuse = length opposite side / sine (26 degrees)
Use Pythagoras's theorem to work out remaining side
and remaining angle = 180 - (90 + 26) = 64 degrees
Question: How do I find the angles of a triangle if I know the lengths of all three sides?
Answer: Use the cosine rule to find one of the angles. You'll need to use the arccos or inverse cos function to work out the value of the angle. Then use the sine rule to find another angle. Finally, use the fact that the sum of the angles is 180 degrees to find the remaining third angle.
Question: What rule would be used to find the length of sides if all three angles are known?
Answer: There is an infinite number of similar triangles that have the same angles. Imagine if you have a triangle and you know all the angles. You can keep making it bigger, but the angles stay the same. However, the sides get longer. So you need to know the length of at least one side. Then you can use the Sine Rule to work out the remaining three sides.
Question: How do you find the side of a right triangle given two angles and hypotenuse?
Answer: If you know two angles, then you can work out the third since all the angles sum to 180 degrees. If the sides are a, b and the hypotenuse is c (opposite angle A), and the angles are A, B and C, then Sin A = a/c, so a = cSin A. Also Cos A = b/c, so b = cCos A.
Question: ABC is a triangle in which AB=20 cm and angle ABC =30°.Given that the area of the triangle is 90 cm^2, find the length of BC ?
Answer: The formula for the area of the triangle is (1/2)AB X BCSinABC
So rearranging:
BC = area / (1/2)ABSin(ABC)
= 2area / ABSin(ABC)
Plug in the values to work out BC:
BC = 2 x 90 / (20 x Sin 30)
Question: How do you solve the side lengths (given only their algebraic values - no numerical ones) and the 90 degree angle?
Answer: Use the sine rule, cosine rule and Pythagoras theorem to express the sides in terms of each other and solve for the unknown variables.
Question: How do you find an angle of an isosceles if you only know two sides and the area?
Answer: Let the triangle have sides of length a, b and c and angles A, B and C.
Angle A is opposite side a
Angle B is opposite side b
Angle C is opposite side c
The two equal sides are a and b and the angle between them is C
Area = (1/2)absinC
a, b and the area are known
So sin C = area / ((1/2)ab)
C = arcsin(area / ((1/2)ab))
A + B + C = 180
But A = B
So A + B + C = 2A + C = 180
So A = (180 - C)/2
Use the cosine rule to find length c
Question: How do I get the area of a scalene triangle if I have two sides and the angle between them?
Answer: Use the formula 1/2abSinC where a and b are the two sides and C is the angle between them.
Question: If I have a 1 length of a triangle and the other angles how do I find the missing length using the sine method?
Answer: Call the sides a, b and c and the angles A, B and C
a is known and also A, B and C
So the sine rule says that a/Sin A = b/Sin B and rearranging gives b = (a/Sin A)Sin B
Similarly a/Sin A = c/Sin C and rearranging gives c = (a/Sin A)Sin C
Question: What is the maximum and minimum value for the sine of an angle?
Answer: If θ is the angle, the maximum value of sine occurs when θ = 90 degrees or π/2 radians. The minimum value is -1 and this occurs when θ = 270 degrees or 3π/2 radians.
Question: A greenhouse can be modeled as a rectangular prism with a half-cylinder on top. The rectangular prism is 20 feet wide, 12 feet high, and 45 feet long. The half-cylinder has a diameter of 20 feet. To the nearest cubic foot, what is the volume of the greenhouse?
Answer: The volume of the rectangular prism section is:
Length x width x height
= 45 x 20 x 12 = 10800 cubic feet
The volume of a cylinder is the cross-sectional area x length
The cross-sectional area is the area of a circle
Let R be the radius = 20/2 = 10
and L be the length = 45
Area = πR²
Volume = πR²L
For a half cylinder
Volume = πR²L/2
= 3.1416 (10)² x 45/2 = 7069 cubic feet to the nearest cubic foot
Total volume = 7069 + 10800 = 17869 cubic feet
Question: How do I know when to use the sine or cosine formula?
Answer: If you know the length of two sides and the angle between them, then you can use the cosine formula to work out the remaining side. Otherwise, the sine formula or Pythagorean theorem can be used.
Question: How should I approach the problem - The triangles ABC and ACD are such that BC- 32 cm, AD - 19cm, CD - 28cm BAC - 74 ( angle ) and ADC - 67 ( angle )?
Answer: Use the cosine rule to work out AC. Then the sine rule to work out the remaining angles/sides.
Question: How do I know when to use sine or cosine formula when given two degrees and one length?
Answer: If the length is opposite one of the known angles, you can use the Sine Rule. If it isn't, you can work out the third angle since the three angles sum to 180 degrees. Then use the Sine Rule. The Cosine Rule is normally used when you only have one angle between two known sides.
Question: Each of the equal angles in an isosceles triangle measures 36 degrees. What is the measure of the third angle?
Answer: All the angles in a triangle add up to 180 degrees. Both angles are 36 degrees so that's 72 degrees. The remaining angle is 180 - 72 = 108 degrees.
© 2016 Eugene Brennan
Eugene Brennan (author) from Ireland on July 03, 2020:
Hi Jacob,
If you two angles, you can calculate the third one because all angles sum to 180 degrees. Then you need at least one side length and you can use the sine rule to calculate the others.
Jacob Halstead on July 03, 2020:
Finding lengths of a trisngle's sides using two base interior angles?
Eugene Brennan (author) from Ireland on June 05, 2020:
Hi Swetha,
You need to know the length of at least one side. There are an infinite number of right angle triangles with the same three angles (similar triangles).
If you know one side, you can use sine and cos to work out the other sides.
Swetha on June 05, 2020:
How to find 3 sides when angles are given in a right angle triangle.Give a formula to solve it?
Eugene Brennan (author) from Ireland on June 02, 2020:
Hi Kayla,
Draw your triangle with the side 8cm as the base. Call this a.
Then draw side c at an angle of 45.5 to side a starting at the left of a. This is angle B. You don't know it's length, so just continue on the line
Draw side b starting at the right of the base a. You don't know the length of b either, so just continue it on to intersect side b.
Use method 2 above for area to first find the length of side c.
So area = 1/2 ac sin B = 1/2 (8) c sin 45.5 = 4c sin 45.5 = 18.54 square cm
Rearranging gives c = 18.54 / (4 sin 45.5)
When you work out this value for c, you can use the cosine rule to find the length of the side b opposite the 45.5 degrees angle. Now you know the lengths of all the sides so you can use the sine rule to work out the angles.
Kayla on June 01, 2020:
Can yu please explain this question?
A triangle has one side length of 8cm and an adjacent angle of 45.5. if the area of the triangle is 18.54cm, calculate the length of the other side that encloses the 45.5 angle
Thanks
Eugene Brennan (author) from Ireland on May 13, 2020:
Hello,
So call the sides a, b and c and the angles A, B and C and assume the sides are a = 5 units, b = 7 units and c = 8 units. It doesn't matter what the actual lengths of the sides are because all similar triangles have the same angles. So if we work out the values of the angles for a triangle which has a side a = 5 units, it gives us the result for all these similar triangles.
Use the cosine rule. So c² = a² + b² - 2abCos C
Substitute for a,b and c giving:
8² = 5² + 7² - 2(5)(7)Cos C
Working this out gives:
64 = 25 + 49 - 70Cos C
Simplifying and rearranging:
Cos C = 1/7 and C = arccos(1/7).
You can use the cosine rule again to find a second angle and the third angle can be found knowing all the angles add to 180 degrees.
Hello on May 13, 2020:
Can I find sinus of the biggest or the smallest angle, if the only thing I know is that the triangle is acute and it's sides are proportional to 5:7:8?
Eugene Brennan (author) from Ireland on May 10, 2020:
Hi Abike,
No, because, there are an infinite number of combinations of angles for the other two angles or two sides.
Draw two lines with the known angle between them. You'll see that you can make the ratio of their lengths anything you want, changing the angles also so that one is big and the other small or vice versa.
Abike on May 10, 2020:
Hello,
Is it possible to find the angles of an acute triangle with only one known angle and no known side?
Eugene Brennan (author) from Ireland on April 29, 2020:
Use the simple formula:
area = 1/2 the base x height
Multiply both sides of equation by 2
2area = 2 x 1/2 x base x height = base by height
Divide both sides by height
2area/height = base x height/height = base
and switch around the two sides
so base = 2area / height.
Suzy on April 28, 2020:
Find the length of the base. Where the height is 8 and the area is 20. Solve for the length base?
Emmy on April 07, 2020:
Thank you so much!
Himanshu gond india on March 12, 2020:
Thanks a lot sir
Eugene Brennan (author) from Ireland on February 27, 2020:
Hi Hassan, if we don't know the length of the side c, we need to know an additional piece of information, the angle between side a and b or one of the other angles.
Hassan on February 27, 2020:
Mr. Brennan, if we have only two side information for example a=5, b=10, and we know nothing about the angles then how to calculate c and any angle. the triangle is not right triangle.
Eugene Brennan (author) from Ireland on February 20, 2020:
No problem Bob, glad to help! Have a great day too!
Bob longnecker on February 20, 2020:
Mr. Brennan
Thank you very much. This is what I was looking for.
Have a great day and best regards .
Bob L.
Eugene Brennan (author) from Ireland on February 20, 2020:
Hi Bob,
The length of the short side is 3.6" x tan(30) which works out at 2.08" approx.
If the angle changes to 31 degrees, the short side is 3.6" x tan(31) = 2.16" approx.
So the length variation of the short side would vary with the tan of the angle. If you look at the graph of tan, there's an approximately linear variation up to about 45 degrees (so the long side increases proportionately with the angle). Then the graph gets steeper at an increasing rate, so the short side would change a lot for small variations of angle.
Bob longnecker on February 18, 2020:
The 3.6 side is opposite the 60° angle. The 3.6 side is the longest of the two short sides. I don't care about the hypotinuse. Just want to really see what a change in the 30° angle does and how it affects the short side. First I need the length of that side and then the length of that side when I change the 30° angle to 31°. How much does 1° change affect the length?
Eugene Brennan (author) from Ireland on February 18, 2020:
In your first problem Bob, which angle is the 3.6" length opposite? (or is this side the hypotenuse, the longest side?)
Bob longnecker on February 17, 2020:
Still trying but no luck!
Eugene Brennan (author) from Ireland on February 17, 2020:
You can also use a triangle calculator like this one and all you have to do is input values for side length and angle. If you have sufficient information, it will calculate the remaining sides and angles.
https://www.calculator.net/triangle-calculator.htm...
Eugene Brennan (author) from Ireland on February 17, 2020:
If the triangle is right angled, then:
sine (angle) = length of side opposite angle / length of hypotenuse
Therefore length of side opposite angle = length of hypotenuse x sine(angle)
Similarly cos (angle) = length of side adjacent to angle / length of hypotenuse.
Therefore length of side adjacent to angle = length of hypotenuse x cos(angle)
Tan(angle) = length of side opposite angle/length of side adjacent.
So if you know all the angles (which you do), and one side, you can work out the remaining sides.
Bob longnecker on February 17, 2020:
Sorry to say I'm 77 years old. I took trig and calc as a senior in high school "60" years ago. Learning it taught me how to think and problem solve in life back then but never used it Perdue after that. Forgot what I learned back then.
Do have a valid reason for the answer just don't have the wear with all to go back and learn trig again.
What I really need to know is how much B changes per degree of change in the hypothesis. Example going from 30 to 31°how much increase in B length ? What is your calculated answer.
Sorry just too tired to go back and visit 60 years ago when I was 17!
Thank you and best regards,
Bob longnecker
Eugene Brennan (author) from Ireland on February 17, 2020:
Hi Bob, you can use the sine, cos and tan relationships to work out problems like this.
Bob longnecker on February 17, 2020:
I have a triangle with angles of: 30,60 and 90°. Side A is know to be 3.6". I want to know what short side B is. Can anyone give me the answer?
Problem #2.
I have a triangle with angles of 31, 59, and 90°. Long side A is 3.6". I want to know the length of short side B.
Hi on February 12, 2020:
solve two triangle and 4 triangle in quadrilateral by use of sine rule
a/sin A= b/sinB that i know
But
a = sin A/ sinB what is that formula
I don't understand that formula but that true
Duran on January 22, 2020:
Hi Mr. Brennan.
I have a problem that is difficult for me:
Known:I have two angles:∠A and ∠B then I have a bundle of similar triangle-ABCs. Now there must be a point T inside the triangles who forms three new sides: TA, TB and TC. I know that the angles between all these three sides are equally 120 deg.
Q: Can I solve the angle-BAT.
That realy confused me for a while !
Eugene Brennan (author) from Ireland on January 04, 2020:
If the angle is 45 degrees, the remaining angle is also 45 degrees, so the triangle is isosceles as well as being right angled. So if the length of the hypotenuse is a and the other two sides are b and c, then from Pythagoras's theorem:
a^2 = (b^2 + c^2) = (2b^2)
so b^2 = (a^2)/2
and b = c = a / square root of 2
Nathaniel Gloyd on January 04, 2020:
If you have a right angle triangle, how would you find the distance from the corner of the 90 degree, to the hypotenuse on a 45 degree angle
Eugene Brennan (author) from Ireland on December 19, 2019:
Hi Rj,
Use the sine rule.
So if your sides are a,b and c and you know their lengths and your angles are A, B and C and you know one angle A, then:
a/sin A = b/sin B
Turn both sides of the equation upside down, so:
sin A / a = sin B / b
Multiply both sides by b
b sin A / a = sin B
Work out b sin A /a on your calculator and this gives you sin B.
Then take the arcsin of the result to get B. Once you have A and B, add together and subtract from 180 to get C.
Rj on December 19, 2019:
If one angle and all three sides of the scalane triangle is given then how will you get the measure of
other two angle
Eugene Brennan (author) from Ireland on October 24, 2019:
Hi Natalia,
Look at method 2 in the tutorial for finding the area of a triangle.
So the area is 1/2 the product of two sides multiplied by the sine of the angle between them.
In your question the sides are PQ and QR and the angle between them is PQR.
So area = (1/2) PQ sin PQR
Substitute for P, Q, angle PQR and the area:
14.2 = (1/2) x 7 x 5 x sin PQR
Rearrange:
sin PQR = 14.2 / ( (1/2) x 7 x 5 )
Take the arcsin of both sides. You can do all this on a calculator, but take care entering all the brackets and numbers because it's very easy to make a mistake. Make sure the calculators is set to "DEG" and use the sin ^ -1 (usually shift on sin) to work out arcsin.
I would recommend HiPer Calc as a good, free scientific calculator app for Android if you have a smartphone.
PQR = arcsin (14.2 / ( (1/2) x 7 x 5 ) ) = 54.235° = 54° 15' approx
natalia on October 24, 2019:
HI EUGENE, can you solve this problem for me and provide me with working out.
the area of triange PQR is 14.2cm squared, find angle PQR to the nearest minute, given PQ is 7cm and QR is 5cm.
Eugene Brennan (author) from Ireland on October 09, 2019:
Hi Pavel,
By diagonal, I presume you mean the hypotenuse.
So you can use Pythagoras' Theorem.
The square on the hypotenuse equals the sum of the squares on the other two sides.
Square the two sides and add together:
(n + 4)² + 16² = (n + 8)²
Expand out:
n² + 8n + 16 + 256 = n² + 16n + 64
Rearrange and simplify:
8n = 208
Giving n = 26
So the two sides are n + 4 = 30 cm and n + 8 = 34 cm
Pavel on October 09, 2019:
I have a problem about a question can you help me please?
I have a right angled triangle the bottom line is 16 cm the one on the side is n+4 and the diagonal line is n+8 can you help me find the two sides please?
Eugene Brennan (author) from Ireland on September 28, 2019:
Hi Carcada. You can't. You can have as many triangles as you want with exactly the same three angles. These are called similar triangles. You need to know at least the length of one side, then you can use the sine rule to work out the others.
Carcada Keischa on September 28, 2019:
if only the angles of each side of the triangle is given then how can we find the length of each side of the triangle?
Eugene Brennan (author) from Ireland on September 08, 2019:
You don't have enough information. You need to have at least one of a, c, A or C.
Sin B = 1/ sqrt 3, only gives you the angle B = (acos (1/sqrt 3)). So if a is the base, side c can be any length without knowing the other sides/angles.
Hannah Adams on September 07, 2019:
I have a question. How do I find the missing sides of a triangle if I know that sin B=1/sqrt 3 and a=2
Eugene Brennan (author) from Ireland on August 14, 2019:
tan (ɵ) = opposite / adjacent so opposite = adjacent x tan (ɵ)
Now you know the opposite and adjacent sidfes, use Pythagoras' theorem to work out the hypotenuse.
Phoebe on August 13, 2019:
Hey, i have a triangle, all that is known is the adjacent, the right angle and the theta, how do i figure out the other sides,
asaba charles on July 23, 2019:
thanks
Maribel Gibbs from Paoli, Pennsylvania on May 22, 2019:
Wow, amazing! One of the best works I ever have seen here!
Khaleel Yusuf on May 18, 2019:
A good review of many years of wining and dining with math calculations. Awesome!
Ur mum gay on April 24, 2019:
This is a decent website
Christopher on March 26, 2019:
Wow this is really helpful thanks
Michael on January 20, 2019:
Hi,
I'm wrapping my head around this problem: I know one side, and the two angles produced by the median on the opposing corner. I'd like to know the length of the other two sides. I drew a scheme, available here:
www.Stavrox.com/image/Triangle.png
The green values are known (a, alpha, beta) , I'd like to calculate b, c and also x. Can you help me.
Ferny Vise from San Francisco, CA on January 19, 2019:
I really like this article. As a math major myself, I believe math is beautiful!
Oscar Skabar on December 02, 2018:
I have an example I cannot work out..... Two birds sitting on a 90 degree mask one at 9m up & the other at 6m up but are 15m apart from each other, they see a fish in the water, how do I calculate the distance of the fish from the birds so they are equal in distance
Rodrigo on November 19, 2018:
Hi, Eugene! You can calc the three angles inside a triangle using tangent half-angle like this:
tan(alpha/2) = r / (p-a)
tan(beta/2) = r / (p-b)
tan(gamma/2) = r / (p-c)
p = (a+b+c) / 2 (semiperimeter)
r = sqrt( (p-a)(p-b)(p-c) / p )
alpha + beta + gamma = 180 (they are the internal angles of the triangle :)
Congrats for your site!
Eugene Brennan (author) from Ireland on November 18, 2018:
Hi Carla. There may be a simpler way of doing it, but you can use the cosine rule in reverse to work out the angle B. Then since it's bisected, you know half this angle. Then use the cosine rule in reverse or the sine rule to work out the angle between sides AB and CA. You know the third angle (between the bisector line and side CA) because the sum of angles is 180 degrees. Finally use the sine rule again to work out the distance from A to the bisection point knowing the length of AB and half the bisected angle.
Eugene Brennan (author) from Ireland on November 05, 2018:
You can't find side lengths with angles alone. Similar triangles have the same angles, but the sides are different. You must have the length of at least one side and two angles.
william on November 05, 2018:
how do you find side lengths with only angle measurements
Eugene Brennan (author) from Ireland on November 03, 2018:
If you have the angle at each end, then you can work out the third angle because you know all the angles add up to 180 degrees. Then use the sine rule to work out each side (see example above in the text)
Karen on November 02, 2018:
i have the the length of one side and the angle at each end, what is the sum to work out the length of the other sides
Eugene Brennan (author) from Ireland on November 02, 2018:
Hi Tom,
If you know the lengths of all three sides, use the cosine rule first and the arccos function to work out one of the angles. Then use the sine rule (or the cosine rule again) to work out the one of the other two angles and the fact that they add up to 180 degrees to find the last angle
As regards Excel, I've added a photo to the article showing how to implement a formula for working out an angle using the cosine rule.
tom sparks on October 23, 2018:
I have a right angled triangle and know the lengths of all three sides. I would like to calculate the other angles.
I have tried TAN in Excel but it says using this 'Returns the tangent of the given angle,.
What would be the best way to work this out
Hope you can help
Kind regards
Eugene Brennan (author) from Ireland on October 21, 2018:
You need more information, either another side or angle to solve.
Sanjeev on October 21, 2018:
Right angle and h is 421.410
How find 2 angles and two sides.
Eugene Brennan (author) from Ireland on September 28, 2018:
You kneed to know at least one other angle or length. The exception is a right-angled triangle. If you know one angle other than the right angle, then you can work out the remaining angles using sine and cos relationships between sides and angles and Pythagoras' Theorem.
SUDHAKAR G on September 28, 2018:
how to i find the length in a Scalene triangle? we konw only one angle and one length.
Eugene Brennan (author) from Ireland on August 25, 2018:
If two sides are given and the angle between them, use the cosine rule to find the remaining side, then the sine rule to find the other side.
If the angle isn't between the known side, use the sine rule to find the angles first, then the unknown side.
You at least need to know the angle between the sides or one of the other angles so in your example it's the sine rule you need to use.
Akhyar on August 24, 2018:
If only two sides are given of a non right angled triangle .. then how to find angle between them
Eugene Brennan (author) from Ireland on July 19, 2018:
Hi Imran,
There's an infinite number of solutions for angles A and B and sides a and B. Draw it out on a piece of paper and you'll see that you can orientate side c with a known length (e.g. pick a length of 10 cm) and change the angles A and B to what ever you want.
You need to know either the length of one more side or one more angle.
Imran Hussain from India on July 19, 2018:
Call the angles A,B and C and the lengths of the sides a, b and c.
a is opposite A
b is opposite B
c is opposite C
C is the right angle = 90º and c is the hypotenuse.
How to find the sides of triangle a and b and other 2 angles A and B, if i know only angle C and side c which is hypotenuse?
Eugene Brennan (author) from Ireland on May 28, 2018:
Hi Liam,
You need to know at least one of the sides.
You could have a very large or very small triangle with the same angles. These are called similar triangles. See the diagram in the tutorial.
Liam on May 27, 2018:
How do I find a side in a right angle triangle if I know all three angles but no sides?
Eugene Brennan (author) from Ireland on May 24, 2018:
If the holes are equally spaced around the imaginary circle, then the formula for the radius of the circle is:
R = B / (2Sin(360/2N))
Where R is the radius
B is the distance between holes
N is the number of holes
Divya on May 24, 2018:
how to calculate distance of each hole at PCD from centre circle
Amar36 on April 17, 2018:
Hi sir
how is that possible to know angle by just having ratios of two heights of triangle and u need not use protector or some other instruments and not even inverse trigonometric functions just simply by ratio do we calculate them or not if then how
I asked it because how they have founded the angles of different triangles with it any discovery of inverse trigonometric functions.
Thank in advance
Eugene Brennan (author) from Ireland on February 13, 2018:
No enough information shahid! If you think about it, there's an infinite number of triangles that satisfy those conditions. Area = (1/2) base x height. So there's no unique values of base and height to satisfy equation (1/2) base x height = 10 m squared.
shahid abbasi on February 13, 2018:
area of right angle triangle is 10m and one angle is 90degree then how calculate three sides and another two angles.
Eugene Brennan (author) from Ireland on January 14, 2018:
If you assign lengths to all sides, you easily can work out the angles. Which sides did assign a length to?
Gem on January 13, 2018:
Any luck Eugene? I have figured out some of the angles by folding a part of the paper that can let me use trig to figure it out if I assign each side a length.
Eugene Brennan (author) from Ireland on January 07, 2018:
Hi Danya,
Because you know two of the angles, the third angle can simply be worked out by subtracting the sum of the two known angles from 180 degrees. Then use the Sine Rule described above to work out the two unknown sides.
danya61 on January 07, 2018:
Hi
I have a triangle with two known angles and one known length of the side between them, and there is no right angle in the triangle. I want to calculate each of unknown sides. How can I do that? (The angle between unknown sides is unknown.)
Eugene Brennan (author) from Ireland on January 04, 2018:
Draw a diagram jeevan. I can't really visualize this.
jeevan on January 04, 2018:
there are 3 circles 1 large circle is a pitch circle having 67 diameter and medium circle is drawn on the circumference of pitch circle at the angle of 5 degree hvaing 11.04 radius and a small circle with only moves in x y direction on pitch circle radius having 1.5 radius so if the medium circle is moved 5degree then at which point the small circle is coinciding and the distance from small circle to center of large/pitch circle.?
sir please help me finding the answer thank you.
Gem on December 29, 2017:
It is tough to prove for sure. I thought I had it by assigning each side a random length ( such as 2cm) and then taking the middle point as half, which looked like the right angle triangle on the top right hand side was half of the half. But it still can't be proven to be half because of the fold.
Eugene Brennan (author) from Ireland on December 16, 2017:
If it's an equilateral triangle, the sides and angles can be easily worked out. Otherwise the triangle can have an infinite number of possible side lengths as the apexes A and C are moved around. So if none of the magnitudes of lengths are known, the expression for lengths of sides of the triangle and its angles would have to be expressed in terms of the square's sides and the lengths AR and CP?
Gem on December 15, 2017:
The whole problem has no measurements or angles. It only has angle names such as A,B,C,D etc. My starting point is from the common knowledge that a square has 4 x 90 degree angles. If I could determine one other angle then I could figure out the whole problem by using the 180 degree rule of triangles. I will snap a picture of it and try and upload it here on Monday, or sketch and upload it. It seems to be a real stumper, 2/70 people at a workshop were able to figure it out, as I was told by the person who passed it along to me. I appreciate your reply, and I look forward to sharing the appropriate visual information with you.
Eugene Brennan (author) from Ireland on December 15, 2017:
Hi Gem,
Is any information given about where the corners of the triangle touch the sides of the square or the lengths of the square's sides? If the triangle isn't equilateral (or even if it is), it seems that there would be an infinite number of placing the triangle in the square.
Gem on December 14, 2017:
Problem: A triangle is placed inside a square. The triangle doesn't have measurements or any listed angles. So we can't identify the type (although it looks equilateral) or make any concrete assumptions about the triangle. I'm suppose to figure out the angles of the triangle without a protractor or ruler based on the only angles I am given which are the 90 degrees from each corner of the square it's in. Since the lines that cut through the square from the main triangle inside the square make new sets of smaller triangles, I still can't make out complimentary or supplementary angles since most of those smaller triangles aren't definitely right angles isosceles triangles.
I'm not sure if my question is clear, so if you answer back I'll try and add a picture or sketch to clarify.
Just picture a square with a triangle in it touching all 3 sides of its points to the square with no units of measure and no angles. We can only assume that the square has 90 degree angles in the corners and that's all we are given to work with.
Thanks Gem
Eugene Brennan (author) from Ireland on December 01, 2017:
Hi Maxy,
Call the angles A,B and C and the lengths of the sides a, b and c.
a is opposite A
b is opposite B
c is opposite C
C is the right angle = 90º and c is the hypotenuse.
If the angle A is known and the side opposite it, a, is known
Then Sin A = opposite/hypotenuse = a/c
So c = a/Sin A
Since you know a and A, you can work out c.
Then use Pythagoras's theorem to work out b
c² = a² + b²
So b² = c² - a²
So b = √(c² - a²)
If the angle A is known and the side adjacent to it, b, is known
Then Cos A = adjacent/hypotenuse = b/c
So c = b / Cos A
Since you know b and A, you can work out c.
Then use Pythagoras's theorem to work out a.
c² = a² + b²
So a² = c² - b²
So a = √(c² - b²)
Eugene Brennan (author) from Ireland on November 27, 2017:
You need to use the cosine rule in reverse.
So if the angles are A, B, and C and the sides are a,b and c.
Then c² = a² + b² - 2abCos C
Rearranging gives angle C = Arccos ((a² + b² - c²) / 2ab)
You can work out the other angles similarly using the cosine rule. Alternatively use the sine rule:
So a/Sin A = c/Sin C
So Sin A = a/c (Sin C)
and A = Arccos ( a/c (Sin C) )
and similarly for the other angles
Hannah on November 27, 2017:
How do you find the angle if all three sides are given
Eugene Brennan (author) from Ireland on November 25, 2017:
Polygons are a lot more complicated than triangles because they can have any number of sides (they do of course include triangles and squares). Also polygons can be regular (have sides the same length) or non-regular (have different length sides).
Here's two formulae:
For a regular or non-regular polygon with n sides
Sum of angles = (n-2) x 180 degrees
For a regular convex polygon (not like a star)
Interior angles = (1 - 2/n) x 180 degrees
Eugene Brennan (author) from Ireland on November 23, 2017:
Hi Jeetendra,
This is called a scalene triangle. The longest edge of any triangle is opposite the largest angle. If all angles are known, the length of at least one of the sides must be known in order to find the length of the longest edge. Since you know the length of an edge, and the angle opposite it, you can use the sine rule to work out the longest edge. So if for example you know length a and angle A, then you can work out a/Sin A.
If c is the longest side,
then a/sin A = c/Sin C ,
so rearranging,
c = a Sin C / Sin A
a, C and A are known, so you can work out c
Jeetendra Beniwal( from India) on November 23, 2017:
If all three angles are given then how we find largest edge of triangle,if all angles are acute
Eugene Brennan (author) from Ireland on July 21, 2016:
Thanks Ron, triangles are great, they crop up everywhere in structures, machines, and the ligaments of the human body can be thought of as ties, forming one side of a triangle.
Ron Bergeron from Massachusetts, US on July 21, 2016:
I've always found the math behind triangles to be interesting. I'm glad that you ended the hub with some examples of triangles in every day use. Showing a practical use for the information presented makes it more interesting and demonstrates a purpose for learning about it.
how to find length of a triangle given one side and angle
Source: https://owlcation.com/stem/Everything-About-Triangles-and-More-Isosceles-Equilateral-Scalene-Pythagoras-Sine-and-Cosine
Posted by: johnsonthearle.blogspot.com

0 Response to "how to find length of a triangle given one side and angle"
Post a Comment